Volume Of A Sphere With Diameter 30 Ft

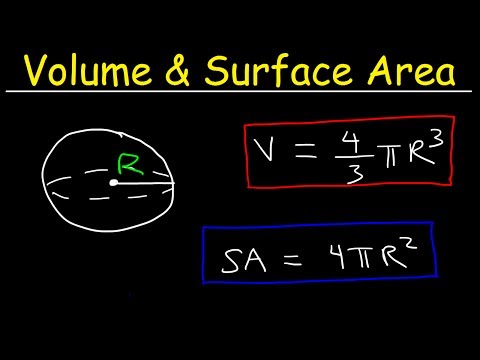
A sphere is a three-dimensional, round object, such as a marble or soccer ball. The volume represents the space enclosed by the object. The formula for the volume of a sphere is 4/3 times pi times the radius cubed. Cubing a number means multiplying it by itself three times, in this case, the radius times the radius times the radius.

To find the volume in terms of pi, leave pi in the formula rather than converting it to 3.14. And to get the volume of a right circular cylinder, you take pi times the radius squared times the height. In other words, to get the surface area of a sphere, you take four times pi times the radius squared. And to get the volume of a sphere, you take the 4/3 of pi times the radius cubed. Enter the diameter of a sphere. The formula for the volume of a sphere is 43 times pi times the radius cubed.

The formula used to calculate the sphere diameter is. This is the diameter of a sphere that corresponds to the specified volume. 17 Find the volume of a sphere with diameter 30 ft. The volume of a sphere is four thirds pi times the radius cubed.

It will also give the answers for volume, surface area and circumference in terms of PI π. A sphere is a set of points in three dimensional space that are located at an equal distance r from a given point . In order to calculate the volume inside a structure, a third dimension needs to be included in the "area" formula. For example, if a circle is given a length or height, it becomes a cylinder and a volume can be calculated.

If a trapezoid or a rectangle has a length it becomes a three-dimensional structure with a volume that can be calculated. A sphere is the shape of a basketball, like a three-dimensional circle. Just like a circle, the size of a sphere is determined by its radius, which is the distance from the center of the sphere to any point on its surface. The formulas for the volume and surface area of a sphere are given below. If you ever wondered what's the volume of the Earth, soccer ball or a helium balloon, our sphere volume calculator is here for you. It can help to calculate the volume of the sphere, given the radius or the circumference.

Also, thanks to this calculator you can determine the spherical cap volume or hemisphere volume. Now the question becomes calculating the volume of the bicylinder . It is also very difficult, so add a cube packing the bicylinder . Now when the plane intersects the cube, it forms another larger square.

The extra area in the large square , is the same as 4 small squares . Moving through the whole bicylinder generates a total of 8 pyramids. Solution for Find the volume of a sphere with a diameter of 30 ft. For any other value for the length of the radius of a sphere, just supply a positive real number and click on the GENERATE WORK button. They can use these methods in order to determine the surface area and volume of parts of a sphere. The formula for the area of a sphere is 4 times 0.785 times the diameter squared.

In other words, to get the area of a circle, you take pi times the radius squared. And to get the circumference of a circle, you take 2 times pi times the radius. The overall diameter of the structure. The calculator applies the diameter to the floor, stem wall, and base of the spherical dome. A sphere with diameter 10 meters is inscribed in a cube What is the volume of the space between the. The volume of a sphere is equal to two -thrid of the volume of a cylinder whose height and diameter.

A set of points in a space equally distanced from a given point $O$ is a sphere. The point $O$ is called the center of the sphere. The distance from the center of a sphere to any point on the sphere is called the radius of this sphere.

A radius of a sphere must be a positive real number. The segment connecting two points on the sphere and passing through the center is called a diameter of the sphere. All radii of the sphere are congruent to each other.

A sphere can be obtained by rotating a semicircle about the diameter. Two spheres of the same radius are congruent. The volume V of a sphere is four-thirds times pi times the radius cubed. As previously stated, circles, rectangles, and trapezoids are the most common shapes in the water industry. However, large standpipes shaped like a cylinder with a sphere on top or an elevated storage tank shaped like a sphere can be very common in the mid-west. Half circles and rectangles can also be found as reservoirs or sedimentation basins.

Therefore, understanding how to calculate the area for these types of structures is also important. Take special note of the units for the diameter. Many times the diameter will be given on some other unit besides feet (e.g. inches). Converting the diameter to feet as your first step will avoid ending up with squared units other than square feet.

Sometimes the diameter of a pipe might be given in metric units. This is common when working with the California Department of Transportation. This statement is not at all obvious or elementary.

"A sphere's volume is two cones of equal height and radius to that of the sphere's". The assertion about the cone and the cylinder is a little easier to prove, but it too is not obvious. So you have not really provided an answer to this to year old question. I think the accepted answer is closest to what you have in mind.

If you want to help here I think you should pay attention to new questions that don't yet have answers. To calculate the volume of a cylinder, you need the radius or diameter of the circular base or top and a cylinder's height. Multiply the radius times the radius. For example, if the radius of your sphere equals 19 inches, multiply 19 by 19 to get 361 square inches. In this calculation you can calculate the volume of a sphere with a number of given input values, such as radius, diameter, circumference. You also have a number of different input units and can choose output unit according to your likings.

Find the volume of a sphere with a diameter of 16 inches. Find the volume of a cone with a height of 114 inches and a radius of 25 inches. Find the surface area of a cone with a height of 19 in and a radius of 9in. Find the volume of a cylinder with height of 90 feet and diameter of 30 feet. A level input measures a slice of the structure. The volume of a sphere formula can be found in terms of the diameter.

The diameter of a sphere is the longest line that is inside the sphere and that passes through the center of the sphere. A sphere is a three-dimensional shape that is perfectly symmetrical and round in shape. Some examples of spheres are a ball, a globe, etc.

The volume of a sphere is the amount of space that is inside it the capacity of the sphere that it can hold. In this article, we will derive the diameter of a sphere formula using the volume. Calculating volume and surface area of sphere play an important role in mathematics and real life as well. Formulas for volume & surface area of sphere can be used to explore many other formulas and mathematical equations. Calculating areas is the first step in working with geometric shapes.

Areas are used to determine how much paint to buy, how much water can flow through a pipe, and many other things. A circle, a rectangle, and a trapezoid are probably the most common shapes you will encounter in the water industry. However, a sphere, a triangle, a half circle with a rectangle can also be found. These are the structures we will focus on in this chapter.

The volume of a cylinder is equal to the product of the area of the circular base and the height of the cylinder. The volume of a cylinder is measured in cubic units. A sphere is the 3- D shape where the curved surface area equals to the total surface area of the figure.

The curved surface area is the area in which only the area of the curved part is covered. The formula does not take into account the circular base. A sphere is a solid figure bounded by a curved surface such that every point on the surface is the same distance from the centre. In other words, a sphere is a perfectly round geometrical object in three-dimensional space, just like a surface of a round ball. Simply enter the desired value in the relevant box.

Please use only numbers (e.g. enter 22 not 22 cm). This calculator only uses numbers. If you try to enter a unit of measure (e.g. 22 metres, 4 miles, 10 cm) you will get an NAN error appear in each box. When you have entered the number that you know, click the button on the right of that box to calculate all the other values.

For example, if you know the volume of a sphere enter the value into the bottom box and then click the calculate button at bottom right. Volume is the amount of total space on the interior of the solid. Knowing the definition of volume, we can now focus on the formulas for volume of common geometric solids. Using these formulas manually won't be difficult, but for fast, accurate results every time, use the volume calculator.

The stem wall height describes a cylinder's height with the floor diameter and the stem wall height. It connects the floor to the dome base. Leave the stem wall height set to zero to remove the stem wall.

All dome structures described by this calculator are a segment or "cap" of a sphere as defined by the dome's diameter and height. The radius of curvature is the calculated radius of the whole sphere of which the dome is a portion. The MDI Spherical Dome Calculator helps calculate common design elements of a partial sphere set on an optional stem wall. It helps with quick design ideas as well as provides accurate measurements when finalizing structural elements.

Outputs include circumference, surface area, volume, and distances along and around the building's various details. The formula used to calculate sphere volume is. SAV A total V. The Volume of sphere given diameter formula is defined as V 43 d2 where d is diameter and V is volume of sphere.

Find the area of the given circle. This tool will calculate the volume of a sphere from the diameter and will convert different measurement units for diameter and volume. A cylindrical glass is 15cm long its diameter is 7cm.

A globe of Earth is in the shape of a sphere with radius 14[/latex] centimeters. Round the answer to the nearest hundredth. Well I wasn't really going for elementary, just a dumbed down version that worked for me that might help others starting at University Math. The other answers were great with backgrounds of why a sphere fits into a cylinder, etc, so I left that part out of mine.

I personally wasn't able to figure out where 4/3 came from using any of their answers, so I contributed mine to help those that may see things my way. I agree it is an old question, but I had this question in 2019 that I couldn't find the answer to with those answers. And to get the volume of a rectangular solid, you take the length times the width times the height. The volume of sphere is the space occupied by it in three dimensional space.

The formula to find the volume is 4/3 π r3. The formula to find the surface area of sphere is 4 times of pi (π) and radius-squared . The total surface area of hemisphere is equal to the sum of its curved surface area and base area . Multiply the result by the radius. In this example, multiply 361 square inches by 19 inches to get 6,859 cubic inches. Simply enter the dimensions into the calculator to find the volume.